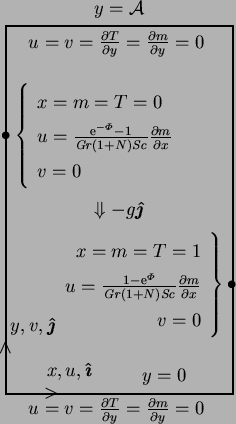
IN CHAPTER 4, the equations of gas-vapour transport were solved for one of the simplest geometries possible: the infinite vertical channel. The usefulness of this solution is limited, of course, by the fact that real cavities are not infinitely tall; they have floors and ceilings. The question here is whether the narrow cavity limiting solution can in fact describe the behaviour of a gas-vapour mixture in a finitely tall cavity, sufficiently far from the floor or ceiling, and in particular, how narrow the cavity must be for this description to be adequate. In this chapter, numerical methods and solutions for the floor and ceiling problems are explained. The assumption of two-dimensionality is retained. The two-dimensional domain is illustrated in figure 5.1.
As pointed out in §4.6.1, the full equations--or at least
those of §4.2--must be solved
in the floor and ceiling regions; the terms inversely proportional to
positive integral powers of
the vertical length scale, 
, can no longer be ignored. This greatly
increases the difficulty of the problem--in particular, the equations are
now nonlinear and strongly coupled--so necessitating a numerical approach.
A significant hydrodynamical difficulty in solving these equations is that the
boundary condition on the velocity at a mass transfer interface
(2.59) depends on the unknown composition gradient.
The flexibility of the finite element package Fastflo (CSIRO 1997)
makes it well suited to the solution of this class of problems.
The first part of this chapter (§§5.1 - 5.3) is devoted to the development of a numerical method for solving the basic equations of vapour transport, after which it is used used to investigate the problem raised in §4.6.1: the floor and ceiling regions.
