The procedure for large ![]() is similar. The solution of (7.20)
as
is similar. The solution of (7.20)
as
![]() is (7.24) but not uniformly in space.
The neglected boundary conditions are at the end-walls. Near the front
end-wall, a coordinate,
is (7.24) but not uniformly in space.
The neglected boundary conditions are at the end-walls. Near the front
end-wall, a coordinate, ![]() , stretched by the factor suggested by
Saint-Venant's principle, is introduced:
, stretched by the factor suggested by
Saint-Venant's principle, is introduced:
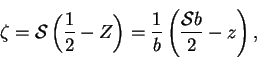 |
(7.55) |
The outer expansion is:
| (7.56) | |||
The inner expansion valid near the front end-wall, ![]() , is:
, is:
| (7.57) | |||
![$\displaystyle \frac{1}{4\upi ^3}\sum_{k=1}^{\infty}\frac{(-1)^k}{k^3}
\sin[(2k+1)\upi X]\mathrm{e}^{-2k\upi \zeta} + o(\mbox{$\mathcal S$}^K),$](img896.png) |
|||
| (7.58) | |||
![$\displaystyle \frac{1}{4\upi ^3} \sum_{k=1}^{\infty}\frac{(-1)^k}{k^3}
\sin(2k\upi X)\exp[-k\upi \mbox{$\mathcal S$}(1-2Z)].$](img899.png) |
This is now a full solution that is practical for large ![]() in the
vicinity of the front end-wall. A solution useful near the back
end-wall is easily constructed by exploiting the even symmetry of
in the
vicinity of the front end-wall. A solution useful near the back
end-wall is easily constructed by exploiting the even symmetry of ![]() with respect to
with respect to ![]() . The first term of (7.59) is the
Jones-Furry solution, the second gives the effect of the front end-wall
and the third the effect of the back end-wall. In the earlier
representation for
. The first term of (7.59) is the
Jones-Furry solution, the second gives the effect of the front end-wall
and the third the effect of the back end-wall. In the earlier
representation for ![]() using the Jones-Furry solution as a particular
integral (7.25), the effects of the two end-walls are
entangled, whereas in (7.59), they are separated.
using the Jones-Furry solution as a particular
integral (7.25), the effects of the two end-walls are
entangled, whereas in (7.59), they are separated.
This representation (7.59) of ![]() , arrived at by physical
reasoning, is identical to that suggested in §7.3.4 on
purely numerical grounds.
, arrived at by physical
reasoning, is identical to that suggested in §7.3.4 on
purely numerical grounds.