| (7.1) |
In the low mass transfer rate limit, the field equations are
(6.16)-(6.19), and the velocity
vanishes on all walls (cf. equation 6.20):
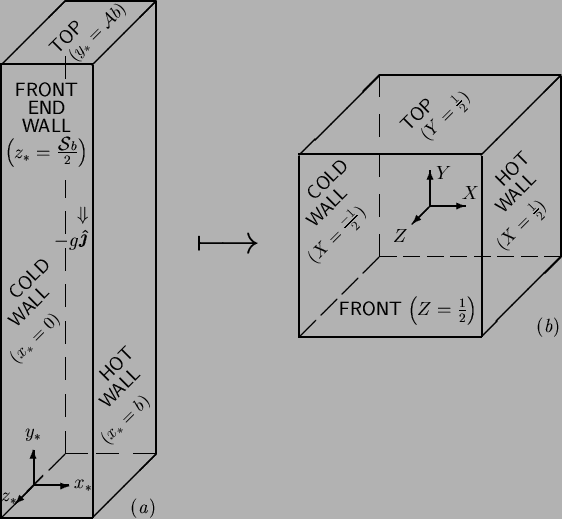
Since Gill's (1966) centrosymmetry properties are regained in the low mass transfer rate limit, it is convenient to translate the origin of the coordinates to the centroid of the cuboid, and, as in chapter 4, use normalized coordinates (see equation 2.74, fig. 7.1b).
 |
A general thermal boundary condition is:
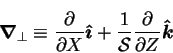 |
(7.3) |
On the end-walls, ![]() leads to a linear temperature variation, while
leads to a linear temperature variation, while
![]() gives an adiabatic condition. On the hot and cold walls,
gives an adiabatic condition. On the hot and cold walls,
![]() implies a uniform heat flux while
implies a uniform heat flux while ![]() gives an isothermal
condition. If the solid presumed to be surrounding the fluid has a
higher thermal conductivity,
gives an isothermal
condition. If the solid presumed to be surrounding the fluid has a
higher thermal conductivity, ![]() everywhere is a simple and consistent
idealization (Batchelor 1954; Leong et al. 1998)
,
although the condition
everywhere is a simple and consistent
idealization (Batchelor 1954; Leong et al. 1998)
,
although the condition ![]() on the heated and
cooled walls and
on the heated and
cooled walls and ![]() on the connecting walls appears frequently in the
literature (Mallinson & de Vahl Davis 1973, 1977; Schladow, Patterson
& Street 1989; Fusegi, Hyun & Kuwahara 1991).
on the connecting walls appears frequently in the
literature (Mallinson & de Vahl Davis 1973, 1977; Schladow, Patterson
& Street 1989; Fusegi, Hyun & Kuwahara 1991).
A boundary condition for ![]() analogous to (7.2) is:
analogous to (7.2) is:
It will be noticed that the reference levels, ![]() and
and ![]() are no
longer evaluated at the cold wall, but are rather taken as the means.
are no
longer evaluated at the cold wall, but are rather taken as the means.